You see an office building. 4 1996

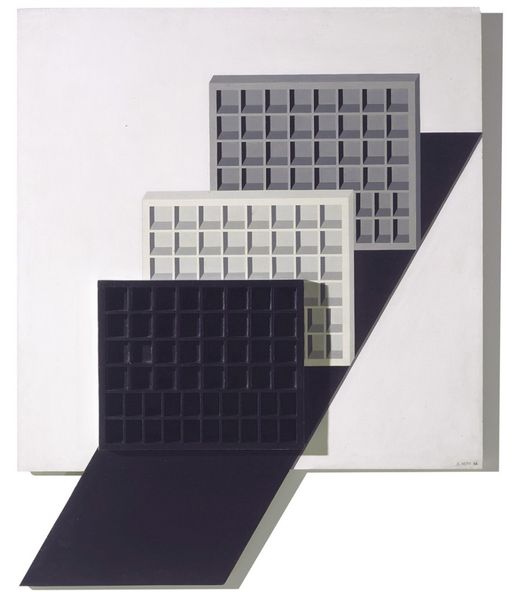
Curator: Julian Opie's piece, "You see an office building. 4," presents a stark, simplified facade. I'm immediately struck by the unsettling calmness of its repetitive geometry. Editor: The depersonalized facade speaks volumes about late capitalism and the erosion of individuality within corporate structures. The building becomes a symbol of uniformity and alienation. Curator: Absolutely, the building's architecture is reduced to its most basic geometric elements, almost hieroglyphic. The window as empty, dark symbols… Editor: Those darkened windows could also be interpreted as vacant stares, reflecting a collective disconnection within the working populace. Or perhaps the windows are symbols of something lurking. Curator: It’s a powerful critique of urban anonymity and the ways in which architecture can both shape and reflect societal power dynamics. This piece really stays with you. Editor: It does. Opie distills the essence of the modern office building, prompting reflection on the narratives of work, identity, and the built environment.